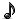The effects of surface elasticity and surface tension on the tra

The effects of surface elasticity and surface tension on the transverse overall elastic behavior of unidirectional nano

-composites

Sofia G. Mogilevskaya ^a^,^,, Steven L. Crouch ^a, Alessandro La Grotta ^b and Henryk K. Stolarski ^a

^a Department of Civil Engineering, University of Minnesota, 500 Pillsbury Drive S.E., Minneapolis, MN 55455, USA

^b Facoltà di Ingegneria, Università di Bologna, Viale Risorgimento 2, 40136 Bologna, Italy

Received 14 August 2009;

revised 4 November 2009;

accepted 11 November 2009.

Available online 16 November 2009.

Abstract

The effects of surface elasticity and surface tension on the transverse overall behavior of unidirectional nano-scale fiber-reinforced composites are studied. The interfaces between the nano-fibers and the matrix are regarded as material surfaces described by the Gurtin and Murdoch model. The analysis is based on the equivalent inhomogeneity technique. In this technique, the effective elastic properties of the material are deduced from the analysis of a small cluster of fibers embedded into an infinite plane. All interactions between the inhomogeneities in the cluster are precisely accounted for. The results related to the effects of surface elasticity are compared with those provided by the modified generalized self-consistent method, which only indirectly accounts for the interactions between the inhomogeneities. New results related to the effects of surface tension are presented. Although the approach employed is applicable to all transversely isotropic composites, in this paper we consider only a hexagonal arrangement of circular cylindrical fibers.

Keywords: A. Nano-composites; A. Fibers; B. Mechanical properties; C. Elastic properties

1. Introduction

The overall (or effective) mechanical behavior of

nano

-scale composite materials is important topic of recent theoretical and experimental investigations [1], [2], [3], [4], [5], [6], [7], [8], [9], [10] and [11]. In such structures, the surface to volume ratio is high and surface effects influence the overall behavior of the materials. The model of elastic surfaces most often employed in recent publications on the topic was developed in the 1970s by Gurtin and Murdoch [1] and [2]. This model includes the concepts of residual surface stresses (surface tension) as well as surface/interface elasticity. Based on Gurtin and Murdoch’s theory, several authors modified classical micromechanical models to account for the surface elasticity. The composite cylinder model and the generalized self-consistent method were used in Duan et al. [3] for analysis of nanoporous materials and in Chen et al. [4] for analysis of

nano

-composites. Duan et al. [3], [5] and [6] modified the composite spheres assemblage model, the generalized self-consistent method, and the Mori–Tanaka method. Chen et al. [7] examined the overall thermoelastic behavior of material using the modified composite spheres assemblage model and the generalized self-consistent method. None of these papers include surface tension in the models. Yang [8] used the concept of dilute concentration to study the influence of surface tension, but the effects of surface elasticity are neglected and an incomplete form of the Gurtin and Murdoch’s model is employed (see [9] for the review of various modifications of the Gurtin and Murdoch’s model used in the literature). In addition, there is a mistake in Yang’s paper leading to an erroneous conclusion that the effective properties of the material depend on the applied strains.

In this work we systematically study the effects of surface elasticity and surface tension on the overall elastic behavior of materials containing aligned long cylindrical nano-inhomogeneities. Materials of this kind are found in practice, e.g. nanochannel-array materials. To evaluate the effective properties, we employ the equivalent inhomogeneity technique proposed in our recent paper [10]. The emphasis of that paper is on the presentation of the method itself and its application to classical problems (including fibers with uniform interphase layers). In the present paper, the same technique is used to systematically study nano-composite systems. First, a finite cluster of nano-inhomogeneities arranged in a pattern representative of the material in question is embedded into an infinite plane with the properties of the matrix and the arbitrary load is applied at infinity. Second, the elastic fields due to the cluster are evaluated based on a semi-analytical solution presented in [9]. Such solution directly and fully accounts for all possible interactions between the nano-inhomogeneities. Finally, a circular inhomogeneity (equivalent inhomogeneity) in an infinite plane is constructed whose size and properties are adjusted so that its effects on the elastic fields at distant points are the same as those of a finite cluster of nano-inhomogeneities. The elastic properties of the equivalent inhomogeneity then define the effective properties of the material. Although the approach employed is applicable to all transversely isotropic composites, in this paper we consider only a hexagonal arrangement of circular cylindrical fibers.

The equivalent inhomogeneity technique described above has some common features with the approach of Maxwell [12] to analyze effective electric conductivity of a particulate composite material. He assumed that spherical particles do not interact with each other and obtained a simple formula for its effective resistance, which is valid only for small volume fractions. Maxell’s approach was recently applied to the prediction of the effective elastic properties of particulate composites in McCartney and Kelly [13]. As in the original Maxell’s approach, the interaction between the particles are not accounted for.

The paper is structured as follows. In Section 2, the problem is formulated, Gurtin and Murdoch’s model is reviewed and two-dimensionless parameters related to the surface elasticity and surface tension are identified. Section 3 is dedicated to comparisons of the results obtained by the equivalent inhomogeneity technique (referred below as EIT) with those available in the literature, which are obtained using the generalized self-consistent method (referred below as GSCM). The comparisons involve the classical case (without surface/interface effects) and the case when the surface elasticity (no surface tension) is included. In Section 4, systematic parametric studies are performed to investigate the effects of both surface elasticity and surface tension on the overall properties of the material with hexagonal arrangement of nano-fibers. Concluding remarks are given in Section 5.

2. Problem formulation. Gurtin and Murdoch’s model

Consider a composite material with a hexagonal arrangement of circular cylindrical nano-fibers aligned in one direction. A two-dimensional plane strain model of an array of N circular inhomogeneities can be used to represent a plane section perpendicular to that direction. Clusters of N=7 and N=19 circular inhomogeneities of equal radii R that represent this material are shown on Fig. 1. We assume that the material of the matrix and that of each fiber is linearly elastic and isotropic. The elastic properties of the nano-inhomogeneities are the same (shear moduli μ_f and Poisson’s ratios ν_f), and in general are different from those of the matrix (μ,ν).

Full-size image (12K)

Fig. 1.

Hexagonal arrays of N=7 and N=19 circular nano-fibers.

The interface between every fiber and the matrix is modeled as a material surface characterized by the surface tension σ₀ and surface elasticity (shear moduli μ₀ and Lamé parameters λ₀) as required by Gurtin and Murdoch’s model. In this model, the displacement are continuous across the matrix/fiber interface, while the stresses undergo a jump governed by the equilibrium equations of that interface

(1)

(2)where σ_kl and σ_kn are the tangential and normal components of the traction vector on the surface of kth fiber, the superscript f (mat) describes the fiber (matrix), s is the arc length of the undeformed surface, is the surface stress, and

(3)

In Eq. (3), u_kl and u_kn are the tangential and normal components of the displacement vector on the surface. The surface stress is defined as [1] and [9]

(4)where is the surface strain given by the following formula

(5)

If the entire system is subjected to a biaxial stress field at infinity , the distributions of stresses, displacements, and strains in the composite system can be determined using a semi-analytical solution presented in [9]. Such solution directly and fully accounts for all possible interactions between the nano-inhomogeneities. The solution is in the form of a series whose coefficients can be found from the system of complex linear algebraic equations (Eqs. (36–38) [9]). The analysis of those equations suggests that—under prescribed stress field at infinity—the solution is governed by the following dimensionless parameters

(6)

The first two parameters are related to the surface/interface properties and the study of their effects on the overall behavior will be emphasized in this work.

3. Comparison of the EIT and GSCM results

3.1. Review of the EIT technique

The basic idea of the EIT [10] is to construct a circular inhomogeneity (equivalent inhomogeneity) in an infinite plane whose size and properties are adjusted so that its effects on the elastic fields at distant points are the same as those of a finite cluster of nano-inhomogeneities arranged in a pattern representative of the material in question. The elastic properties of the equivalent inhomogeneity define the effective properties of the material and can be determined from algebraic formulae, provided that the stresses outside of the cluster are known. The volume ratio of the composite material is not given a priori but is found in the course of the solution procedure. More details about the technique are given in the Appendix A.

In [10] we presented extensive comparison of the results obtained by this technique with benchmark exact analytical and numerical solutions available for classical problems (γ=χ=0) and showed that the technique works equally well for periodic and random composites. No comparison with the predictions by the classical micromechanical models was presented in that paper. In the meantime, the only available results for nano-scale fiber-reinforced composites (related to the effects of surface elasticity only) have been obtained using the modified micromechanical models developed by Duan et al. [3] and Chen et al. [4]. Thus, we begin this study by presenting the comparison of the results obtained by EIT approach with those by GSCM both for the classical case (without surface/interface effects) and the case when the surface elasticity (no surface tension) is included.

3.2. Comparison for the classical case (γ=χ=0)

To get better understanding of the subsequent results, we first consider the reference (classical) case and compare the results predicted by the EIT with those obtained by the classical GSCM (also known as the three phase cylinder model, Christensen and Lo [14]). It is well-known that the prediction of the latter model for the effective two-dimensional bulk modulus coincides with that of the composite cylinder model.

We consider the case of hollow fibers (μ_f=0,ν_f=0). For this case, the GSCM provides the following analytical expression for the normalized two-dimensional plane strain effective bulk modulus

(7)where f is the porosity and k is a two-dimensional bulk modulus of the matrix related to its three-dimensional counterpart K by the formulae k=K+μ/3.

The normalized effective shear modulus μ_eff/μ (two- and three-dimensional shear moduli are the same) is found as a positive solution of the following quadratic equation

A(μ_eff/μ)²+2B(μ_eff/μ)+C=0where

(8)A=-3f(1-f)²-(κ+f³)(κf+1)

For the case of hollow fibers, the following asymptotic (high concentration) results were proposed by Day et al. [15]

(9)

where ν is two-dimensional Poisson’s ratio related to its three-dimensional counterpart ν⁽³⁾ by the formulae ν=ν⁽³⁾/(1-ν⁽³⁾) and f_c is the critical volume fraction that corresponds to the case when the fibers touch (the so-called percolation threshold). The value of f_c for our geometrical configuration (Fig. 1) is . As can be seen from expressions (7) and (8), the GSCM method provides the percolation threshold f_c=1, independently of the geometrical arrangement of the fibers, which reflects approximate character of the model.

We evaluated the overall properties of perforated anodic alumina (shear modulus and Poisson’s ratio ν=0.3). All results presented here were obtained using a representative pattern with N=19 nano-fibers shown in Fig. 1. This decision was made based on some benchmark calculations done with and N=37, which showed that, while there was a very small difference between the results obtained with N=7 and N=19, the results obtained with N=19 and N=37 were indistinguishable from one another.

Fig. 2 and Fig. 3 demonstrate the comparison of our results for the normalized two-dimensional bulk (Fig. 2) and shear (Fig. 3) moduli as functions of porosity f with those obtained by the GSCM. Asymptotic results by Day et al. [15] and the Hashin–Shtrikman upper bound [16] are also shown on the figures; for the effective bulk modulus GSCM and the Hashin–Shtrikman upper bound yield the same results. Lower Hashin–Shtrikman bound for both moduli is zero. Interestingly, the Hashin–Shtrikman upper bounds for the effective bulk and shear moduli coinside with the results obtained by McCartney and Kelly [13] using Maxell’s [12] approach.

Full-size image (14K)

Fig. 2.

Normalized effective two-dimensional bulk modulus vs. porosity (classical case).

Full-size image (19K)

Fig. 3.

Normalized effective shear modulus vs. porosity (classical case).

One can see from Fig. 2 and Fig. 3 that while the predictions by the GSCM and EIT for the effective bulk modulus are close for a wide range of porosities, the predictions for the effective shear modulus by those methods are quite different starting with the porosities that are higher than about 0.2. We believe that our approach is more accurate as it fully accounts for all possible interactions between the fibers. The comparison with high concentration results seems to support such conclusion.

3.3. Comparison for the case with surface elasticity

We compare our results with the those by Chen et al. [4] obtained by the modified GSCM. The latter method provides the following analytical expression for the normalized two-dimensional plane strain effective bulk modulus (Eq. (3.2) from [4]).

(10)

This expression is consistent with the one presented in Duan et al. [3] (Eq. (26) in that paper). The expression for the normalized effective shear modulus provided by the modified GSCM can be obtained from [4] (Eqs. (B1)–(B5)).

For the sake of comparison, we used the same material property of alumina and sets of surface elasticity parameters ( and ) as the ones presented in [4]. Fig. 4 and Fig. 5 show the comparison of the results for the normalized two-dimensional bulk (Fig. 4) and shear (Fig. 5) moduli as functions of porosity f for two sets of dimensionless parameter γ(they correspond to the results for and sets A and B of surface elasticity parameters used in [4]). Just as those of Chen et al. [4], our results are normalized by the corresponding values for the classical case (without surface/interface effects).

Full-size image (14K)

Fig. 4.

Normalized effective two-dimensional bulk modulus vs. porosity: solid line – EIT, dots – GSCM (μ_f/μ=0,χ=0).

Full-size image (15K)

Fig. 5.

Normalized effective shear modulus vs. porosity: solid line – EIT, dots – GSCM (μ_f/μ=0,χ=0).

We can see from Fig. 4 and Fig. 5 that the results predicted by the EIT demonstrate more pronounced influence of surface elasticity on the effective two-dimensional bulk modulus and less pronounced influence on the effective shear modulus than anticipated by the modified GSCM. As in the classical case, the EIT and the modified GSCM approaches are in better agreement in predicting the effective bulk modulus than the effective shear modulus.

No numerical results for the effective transverse Poisson’s ratio have been reported in [3] and [4]. In EIT approach, the effective Poisson’s ratio is computed naturally and easily in the same manner as other in-plane effective materials constants. Nonetheless, all those independently obtained two-dimensional material constants (bulk and shear moduli as well as Poisson’s ratio) satisfy the well-known relations with high degree of accuracy and this constitutes yet another test for the validity of the EIT. The normalized two-dimensional effective Poisson’s ratios obtained by the EIT are plotted in Fig. 6 as functions of porosity. The results demonstrate a pronounced influence of surface elasticity on the two-dimensional effective Poisson’s ratio for porosities larger than about f=0.5.

Full-size image (11K)

Fig. 6.

Normalized effective two-dimensional Poisson’s ratio vs. porosity (μ_f/μ=0,χ=0).

4. Parametric studies

In this Section, the effects of surface elasticity and surface tension on the overall properties of the material with a hexagonal arrangement of nano-fibers are systematically studied. We again use the material properties of alumina, and ν=0.3, and vary the parameters γ and χ given by Eq. (6). The considered values of these parameters are consistent with the surface properties of alumina reported in Miller and Shenoy [17]; Gurtin and Murdoch [2]; and Sharma and Ganti [18].

4.1. Influence of surface elasticity

We first consider the case of perforated material (μ_f/μ=0). Fig. 7 and Fig. 8 show the results for the normalized two-dimensional bulk (Fig. 7) and shear (Fig. 8) moduli as functions of porosity f for several sets of dimensionless parameter γ.

Full-size image (16K)

Fig. 7.

Normalized effective two-dimensional bulk modulus vs. porosity (μ_f/μ=0,χ=0).

Full-size image (16K)

Fig. 8.

Normalized effective shear modulus vs. porosity (μ_f/μ=0,χ=0).

The analysis of the figures suggests that, as expected, the material surfaces characterized by positive values of γ increase the overall stiffness of nano-composites, while the surfaces characterized by negative values of γ decrease it. More importantly, the increase or decrease in stiffness can be quite significant for materials characterized by large absolute values of γ, even for relatively modest values of porosity.

Second, we consider materials with stiff nano-inhomogeneities (μ_f/μ=2,ν_f=ν). Fig. 9 and Fig. 10 show the results for the normalized two-dimensional bulk (Fig. 9) and shear (Fig. 10) moduli as functions of porosity f for the same sets of dimensionless parameter γ as those used above. It can be seen that the influence of surface elasticity diminishes in this case as compared with the case of perforated materials.

Full-size image (15K)

Fig. 9.

Normalized effective two-dimensional bulk modulus vs. volume fraction (μ_f/μ=2,χ=0).

Full-size image (15K)

Fig. 10.

Normalized effective shear modulus vs. volume fraction (μ_f/μ=2,χ=0).

4.2. Influence of surface tension

The presence of surface tension is associated with residual stresses and deformations everywhere within the composite system even if no external load is applied. Thus, to evaluate the effective properties properly, those residual effects need to be eliminated from the solution due to the applied load. To do so, the problem of the cluster must be solved twice, once with both the external load and surface tension included and the second time with just surface tension. The second solution is subtracted from the first one and the resulting elastic fields away from the cluster are processed as in the case without surface tension (see Appendix A). Such a two-step approach accurately represents the physics of the problem and is critical to evaluate the effective properties correctly. Without it, an erroneous result may be obtained indicating that, even with the framework of linear elasticity, the effective elastic properties are strain dependent (Yang [8]).

We consider a case of perforated material (μ_f/μ=0). We have found that surface tension has negligible effects on the two-dimensional effective bulk modulus. For this reason, the normalized results are trivial and not presented here. Fig. 11 and Fig. 12 show the results for the normalized shear modulus (Fig. 11) and the normalized two-dimensional Poisson’s ratio (Fig. 12) as functions of porosity f for several sets of dimensionless parameter χ.

Full-size image (12K)

Fig. 11.

Normalized effective shear modulus vs. porosity (μ_f/μ=0,γ=0).

Full-size image (13K)

Fig. 12.

Normalized effective two-dimensional Poisson’s ratio vs. porosity (μ_f/μ=0,γ=0).

We can conclude that surface tension seems to increase the normalized effective shear modulus while decreasing the two-dimensional effective Poisson’s ratio.

4.3. Combined influence

Consider again a case of a perforated material (μ_f/μ=0) and assume the following sets of values of the dimensionless parameters: γ=0.08777,χ=0.03112. These values were selected based on the previous results, which showed that each parameter separately amplified the overall properties. In this case the difference is significant enough to be discernible on a graph presenting values normalized in a different way than in previous figures. In Fig. 13 and Fig. 14, the results are normalized with respect to those of the material matrix, which are independent of porosity, while non-normalized values of Poisson’s ratio are plotted in Fig. 15. The reference classical case (γ=0,χ=0) is plotted in all figures for the comparison. From Fig. 13 and Fig. 14 we conclude that combined effects of surface elasticity and surface tension on the two-dimensional effective bulk modulus is somewhat smaller than on the effective shear modulus. We attribute this to the fact that, as observed above, the effects of surface tension on the two-dimensional effective bulk modulus are negligible. The effects of surface elasticity and surface tension on the effective shear modulus are both present and amplify one another. Fig. 15 reveal a rather dramatic influence of combined surface effects on the effective two-dimensional Poisson’s ratio, particularly for highly porous materials.

Full-size image (15K)

Fig. 13.

Normalized effective two-dimensional bulk modulus vs. porosity.

Full-size image (15K)

Fig. 14.

Normalized effective shear modulus vs. porosity.

Full-size image (12K)

Fig. 15.

Effective two-dimensional Poisson’s ratio vs. porosity.

5. Concluding remarks

In this paper, we present comprehensive studies of the influence of surface elasticity and surface tension on the overall elastic transverse properties of nano-composite materials based on Gurtin and Murdoch’s model [1] and [2]. The studies were conducted using an equivalent inhomogeneity technique that has several unique, important, and powerful features:

(a) it is based on the complete Gurtin and Murdoch model;

(b) it fully accounts for all possible interactions between the nano-inhomogeneities; and

(c) all transverse effective properties are obtained from the analysis of a single problem involving one type of a load at infinity, e.g. uniaxial load. Those properties are related to each other by the standard formulae.

The results reveal that:

(a) For the bulk modulus, the GSCM provides quite accurate results for a large range of volume fractions. However, for the shear modulus, the results obtained via the GSCM are less accurate starting with the volume fractions that are higher than about 0.2. The GSCM does not directly predict Poisson’s ratio. It is possible to obtain Poisson’s ratio from the bulk and shear moduli, but—given the inaccuracies in shear modulus—such an approach would lead to inaccurate values for Poisson’s ratio. In our approach, all three constants are computed independently and they satisfy the well-known inter-relationships with high accuracy.

(b) The material surfaces characterized by positive values of parameter γ, which specifies the magnitude of surface elasticity, increase overall stiffness of nano-composites, while the surfaces characterized by negative values of γ decrease it. The influence of surface elasticity diminishes in the case of composite materials containing solid nano-inhomogeneities as compared with the case of perforated materials.

(c) The surface tension has a negligible effect on the two-dimensional effective bulk modulus. It increases the effective shear modulus while decreasing the two-dimensional effective Poisson’s ratio.

(d) Even though separate effects of surface tension and surface elasticity may not be that pronounced, we have shown that for some values of surface parameters, their combined influence may be rather dramatic, particularly for highly porous materials.

We would like to emphasize that the results presented in this work have been obtained using the ranges of surface parameters that were reported in the literature and characteristic of only some particular materials. It is reasonable to expect that there exist (or can be designed) materials for which the surface parameters are such that their effects will be even more significant.

References

[1] M.E. Gurtin and A.I. Murdoch, A continuum theory of elastic material surfaces, Arch Ration Mech Anal 57 (1975), pp. 291–323. View Record in Scopus | Cited By in Scopus (282)

[2] M.E. Gurtin and A.I. Murdoch, Surface stress in solids, Int J Solids Struct 14 (1978), pp. 431–440. Abstract | PDF (750 K) | View Record in Scopus | Cited By in Scopus (89)

[3] H.L. Duan, J. Wang, B.L. Karihaloo and Z.P. Huang, Nanoporous materials can be made stiffer that non-porous counterparts by surface modification, Acta Mater 54 (2006), pp. 2983–2990. Article | PDF (401 K) | View Record in Scopus | Cited By in Scopus (34)

[4] T. Chen, G.J. Dvorak and C.C. Yu, Size-dependent elastic properties of unidirectional nano-composites with interface stresses, Acta Mech 188 (2007), pp. 39–54. Full Text via CrossRef | View Record in Scopus | Cited By in Scopus (30)

[5] H.L. Duan, J. Wang, Z.P. Huang and B.L. Karihaloo, Size-dependent effective elastic constants of solids containing nano-inhomogeneities with interface stress, J Mech Phys Solids 53 (2005), pp. 1574–1596. Article | PDF (413 K) | View Record in Scopus | Cited By in Scopus (127)

[6] H.L. Duan, X. Yi, Z.P. Huang and J. Wang, A unified scheme for prediction of effective moduli of multiphase composites with interface effects. Part I: theoretical framework, Mech Mater 39 (2007), pp. 81–93. Article | PDF (253 K) | View Record in Scopus | Cited By in Scopus (17)

[7] T. Chen, G.J. Dvorak and C.C. Yu, Solids containing spherical nano-inclusions with interface stresses: effective properties and thermal–mechanical connections, Int J Solids Struct 44 (2007), pp. 941–955. Article | PDF (321 K) | View Record in Scopus | Cited By in Scopus (16)

[8] F.Q. Yang, Size-dependent effective modulus of elastic composite materials: spherical nanocavities at dilute concentrations, J Appl Phys 95 (2004), pp. 3516–3520. Full Text via CrossRef | View Record in Scopus | Cited By in Scopus (52)

[9] S.G. Mogilevskaya, S.L. Crouch and H.K. Stolarski, Multiple interacting circular nano-inhomogeneities with surface/interface effects, J Mech Phys Solids 56 (2008), pp. 2298–2327. Article | PDF (3474 K) | View Record in Scopus | Cited By in Scopus (18)

[10] S.G. Mogilevskaya, S.L. Crouch, H.K. Stolarski and A. Benusiglio, Equivalent inhomogeneity method for evaluating the effective elastic properties of unidirectional multi-phase composites with surface/interface effects, Int J Solids Struct 47 (2010), pp. 407–418. Article | PDF (898 K) | View Record in Scopus | Cited By in Scopus (2)

[11] L. Tian and R.K.N.D. Rajapakse, Analytical solution for size-dependent elastic field of a nanoscale circular inhomogeneity, J Appl Mech (2007), pp. 568–574. Full Text via CrossRef | View Record in Scopus | Cited By in Scopus (15)

[12] J.C. Maxwell, A treatise on electricity and magnetism (1st ed.), Clarendon Press, Oxford (1873) vol. 1, p. 435–41.

[13] L.N. McCartney and A. Kelly, Maxwell’s far-field methodology applied to the prediction of properties of multi-phase isotropic particulate composites, Proc Roy Soc A 464 (2008), pp. 423–446. Full Text via CrossRef | View Record in Scopus | Cited By in Scopus (5)

[14] R.M. Christensen and K.H. Lo, Solutions for effective shear properties in three phase sphere and cylinder models, J Mech Phys Solids 27 (1979), pp. 315–330. Abstract | PDF (980 K) | View Record in Scopus | Cited By in Scopus (626)

[15] A.R. Day, K.A. Snyder, E.J. Garboczi and M.F. Thorpe, The elastic moduli of a sheet containing circular holes, J Mech Phys Solids 40 (1992), pp. 1031–1051. Abstract | PDF (1409 K) | View Record in Scopus | Cited By in Scopus (85)

[16] Z. Hashin and S. Shtrikman, A variational approach to the theory of the elastic behaviour of multiphase composites, J Mech Phys Solids 11 (1963), pp. 127–140. Abstract | PDF (889 K) | View Record in Scopus | Cited By in Scopus (1270)

[17] R.E. Miller and V.B. Shenoy, Size-dependent elastic properties of nanosized structural elements, Nanotechnology 11 (2000), pp. 139–147. Full Text via CrossRef | View Record in Scopus | Cited By in Scopus (330)

[18] P. Sharma and S. Ganti, Interfacial elasticity corrections to size-dependent strain-state of embedded quantum dots, Phys Status Solidi 234 (2002), pp. R10–R12. Full Text via CrossRef | View Record in Scopus | Cited By in Scopus (35)

Appendix A. Equivalent inhomogeneity technique

We consider two problems shown in Fig. 16a and b. Fig. 16a contains a cluster of N circular nano-inhomogeneities arranged in a pattern representative of the composite material. The cluster is embedded in an infinite plane with the properties of the material matrix and the arbitrary (e.g. uniaxial) load is applied at infinity. Contour L in Fig. 16a is the boundary of a circle with center that coincides with the center of the cluster and whose radius r is large enough to surround the cluster. The complex tractions σ(z)=σ_n(z)+iσ_s(z), (where σ_n(z) and σ_s(z) are the normal and shear components and z=x+iy) at the boundary L due to the cluster are evaluated based on a semi-analytical solution presented in [9].

Full-size image (14K)

Fig. 16.

(a) A cluster of circular inhomogeneities in an infinite plane. (b) The equivalent circular inhomogeneity.

Fig. 16b contains only one (equivalent) inhomogeneity whose properties coincide with the effective properties, μ_eff,ν_eff of the composite material. The exact expression for σ(z) at the same boundary L in this case is as follows:

(11)where

(12)and the coefficients A_-2(r),A₀(r),A₂(r) involved in expression (11) are dependent on the properties of that inhomogeneity and its radius.

The basic idea of the EIT is that the tractions at the boundary L for these two problems are the same. Thus, representing the solutions for σ(z) due to the cluster (Fig. 16a) in the form of expression (11), one can find the coefficients A_-2(r),A₀(r),A₂(r). From those coefficients one can calculate the effective properties μ_eff,ν_eff,k_eff and the radius R_eff, as explained in [10]. The volume fraction is found from the following expression

(13)

As described in [10], the choice of the radius r for the contour L within the interval (2R_cls,100R_cls),R_cls is the radius of the smallest circle that contains the cluster, provides accurate values of the effective properties.

Corresponding author. Tel.: +1 612 625 4810; fax: +1 612 626 7750.

مدیر سایت 1389,11,30 ساعت 13:30

Free Textile Article

Free Textile Article

درباره من